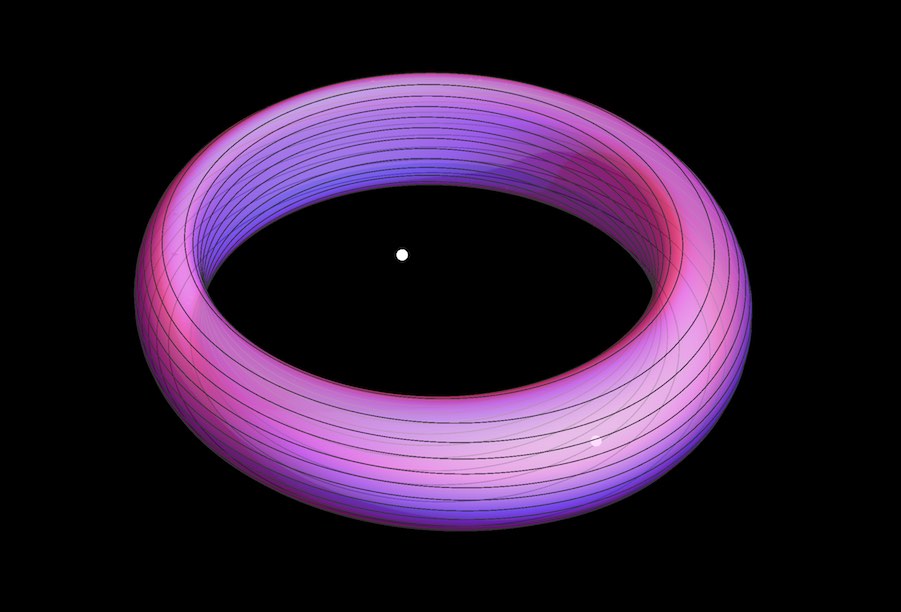
Monodromy in Hamiltonian systems has been traditionally associated to singularities of the integrable Hamiltonian fibration and, in particular, to singularities of focus-focus type. In a recent work with Nikolay Martynchuk, we showed that in 2 degree of freedom Hamiltonian systems with a circle action, to understand monodromy, one should look at the fixed point of the circle action. This result follows from the fact that, in such systems, a 2-torus bundle $E$ over a circle $\Gamma$ can be also viewed as a principal circle bundle over a 2-torus $B$. Then the monodromy of the former bundle equals the Chern number of the latter.
This is neatly shown in the following picture where I have drawn $B$ for the 2-torus bundle over a closed path $\Gamma$ going around the focus-focus critical value in the spherical pendulum. One of the two fixed points of the circle action (shown in white) lies “inside” $B$ and this induces a non-zero Chern number for the principal circle bundle over $B$ and thus a non-trivial monodromy for the 2-torus bundle over $\Gamma$. Details can be found in our paper which will appear in the Journal of Geometry and Physics.
Moreover, our result can be generalized to integrable Hamiltonian systems with $n$ degrees of freedom and a $(n-1)$-torus action. In this case we give a simple formula for determining monodromy based on the number of singular orbits of the action with circle isotropy.